Exploring The Fascinating World Of 10 Cubed

Mathematics is a realm of endless wonders, and among its many captivating aspects is the concept of cubing numbers. One such number that holds a special place in this mathematical universe is 10. Cubing 10, or "10 cubed," results in a significant value that has practical applications in various fields, from science to engineering. This article delves into the intricacies of 10 cubed, uncovering how this simple operation can yield remarkable insights and applications.

The operation of cubing a number involves multiplying it by itself twice. Therefore, 10 cubed translates to 10 multiplied by 10 and then by 10 again. The result of this operation is a powerful 1,000, which opens the door to understanding volumes, areas, and even larger mathematical concepts. This article seeks to demystify the significance of 10 cubed, including its relevance in real-world scenarios and its relationship with other mathematical principles.

As we explore the concept of 10 cubed, we will address various questions surrounding it, such as: What are the applications of 10 cubed in different fields? How can we visualize this concept in practical terms? What role does 10 cubed play in everyday life? By answering these questions, we aim to provide a comprehensive understanding of the importance of 10 cubed and its broader implications.

What is the Mathematical Calculation of 10 Cubed?

To calculate 10 cubed, we follow the mathematical operation of exponentiation. In mathematical terms, cubing a number means raising it to the power of three. So, for 10 cubed, the calculation is as follows:

10 cubed = 10 × 10 × 10 = 1,000

This simple arithmetic operation can generate significant insights, especially when considering its applications in geometry, physics, and other scientific disciplines.

How is 10 Cubed Used in Real Life?

The value of 1,000, derived from 10 cubed, has numerous practical applications across various fields. Here are a few examples:

• Volume Calculations: In geometry, 10 cubed is often used to determine the volume of a cube with a side length of 10 units. The volume of such a cube would be 1,000 cubic units.
• Data Analysis: In data science, the concept of cubing can be utilized to analyze data sets, particularly when dealing with large quantities of information.
• Physics: Cubing numbers often appears in physics equations, such as those related to density, where volume plays a crucial role.

What are the Mathematical Properties of 10 Cubed?

Understanding the properties of 10 cubed can deepen our appreciation for this mathematical operation. Here are some significant properties:

• Associative Property: The result of 10 cubed is independent of how the numbers are grouped in multiplication.
• Commutative Property: The order in which numbers are multiplied does not affect the final product.
• Identity Property: Any number multiplied by 1 remains unchanged.

What Are Some Fun Facts About 10 Cubed?

Beyond its mathematical significance, 10 cubed has some intriguing fun facts associated with it:

• Base of the Decimal System: The number 10 is foundational to our decimal number system, which is based on ten digits: 0 through 9.
• Cultural Significance: The number 10 is often associated with completeness or perfection in various cultures and contexts.
• Power of Three: Cubing a number is a powerful operation, and 10 cubed exemplifies this by representing a substantial quantity.

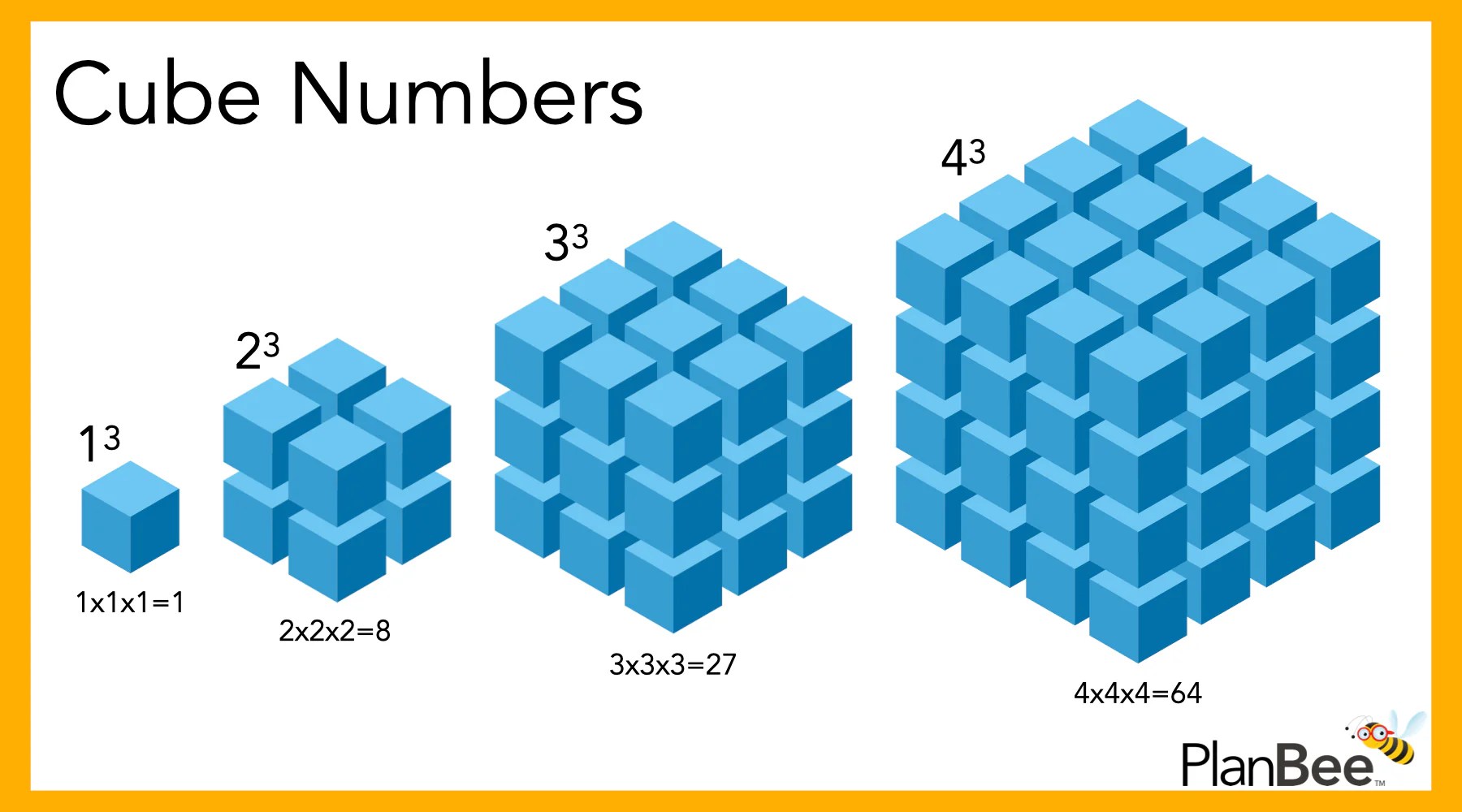
How Can We Visualize 10 Cubed?

Visualizing 10 cubed can be immensely helpful in comprehending its significance. One way to visualize this concept is through geometric shapes:

• Imagine a cube with each side measuring 10 units. The volume of this cube would be represented by 10 cubed, or 1,000 cubic units.
• Another visualization could be stacking 10 rows of 10 blocks, resulting in a total of 100 blocks. If we were to stack these rows 10 high, we would reach 1,000 blocks.

What is the Relationship Between 10 Cubed and Other Powers?

To further understand 10 cubed, it is essential to explore its relationship with other powers of 10:

• 10 to the Power of 1: 10^1 = 10
• 10 to the Power of 2: 10^2 = 100
• 10 to the Power of 3: 10^3 = 1,000 (10 cubed)
• 10 to the Power of 4: 10^4 = 10,000

This pattern indicates that as we increase the exponent, the value grows exponentially, showcasing the power of cubing and higher powers of 10.

How Does 10 Cubed Relate to Other Mathematical Concepts?

10 cubed serves as a foundation for various mathematical concepts, including:

• Exponents: Understanding 10 cubed helps solidify knowledge of exponents and their role in mathematics.
• Volume: The concept of cubing is fundamental in calculating volumes of three-dimensional shapes.
• Arithmetic Operations: Mastering cubing enhances overall arithmetic skills and the ability to tackle more complex mathematical problems.

Can 10 Cubed Be Applied in Technology?

Indeed, the concept of 10 cubed finds its applications in technology as well:

• Computing: In computer science, cubing can be applied to algorithms that deal with three-dimensional data.
• Simulations: Engineers often use cubed measurements in simulations for designing structures and systems.
• Data Storage: The capacity of storage devices is often measured in cubic units, making 10 cubed relevant in this context.

How Can We Teach 10 Cubed to Students?

Teaching the concept of 10 cubed can be engaging and insightful. Here are some strategies:

• Hands-On Activities: Use blocks or cubes to allow students to physically manipulate and visualize the concept of cubing.
• Real-World Applications: Highlight real-life scenarios where cubing is relevant, such as building projects or data analysis.
• Games and Challenges: Create math challenges that focus on cubing to make learning fun and interactive.

In summary, 10 cubed is a fundamental mathematical concept that extends beyond numbers. Its applications span various fields, making it an essential part of understanding mathematics and its relevance in our daily lives. Whether in geometry, physics, or technology, the significance of 10 cubed is undeniable, showcasing the power of mathematics in shaping the world around us.

Also Read

Article Recommendations

ncG1vNJzZmivp6x7tMHRr6CvmZynsrS71KuanqtemLyue9OrsJ6bmKR%2FcXuQaWScrZKasW%2B006aj