How To Find The Missing Side Of A Triangle

Publish date: 2024-10-21

Understanding triangles is a fundamental aspect of geometry that can unlock many mathematical concepts and real-world applications. Whether you're a student grappling with homework or an adult looking to refresh your knowledge, knowing how to find the missing side of a triangle is an essential skill. Triangles come in various types, each with unique properties that can simplify the process of calculating unknown sides. This article will guide you through the various methods to find the missing side of a triangle, ensuring you grasp the concepts thoroughly.

In geometry, a triangle is defined by its three sides and three angles. The relationship between these sides and angles is governed by the properties of triangles, such as the Pythagorean theorem and the sine, cosine, and tangent functions. It's crucial to understand these relationships to correctly find the missing side of a triangle, especially when working with right triangles or using trigonometric methods. By the end of this article, you'll be equipped with the knowledge to tackle any triangle problem with confidence.

Whether you are calculating dimensions for construction, designing graphics, or solving mathematical problems, being proficient in how to find the missing side of a triangle can be incredibly beneficial. In this comprehensive guide, we will break down the steps and formulas needed to determine the unknown side, providing real-life examples to help solidify your understanding.

What is a Triangle?

A triangle is a polygon with three edges and three vertices. The sum of the interior angles of a triangle is always 180 degrees. There are several types of triangles, including:

Equilateral Triangle: All sides and angles are equal.
Isosceles Triangle: Two sides are of equal length, and the angles opposite those sides are equal.
Scalene Triangle: All sides and angles are different.
Right Triangle: One angle is exactly 90 degrees.

How Does the Pythagorean Theorem Help in Finding the Missing Side of a Triangle?

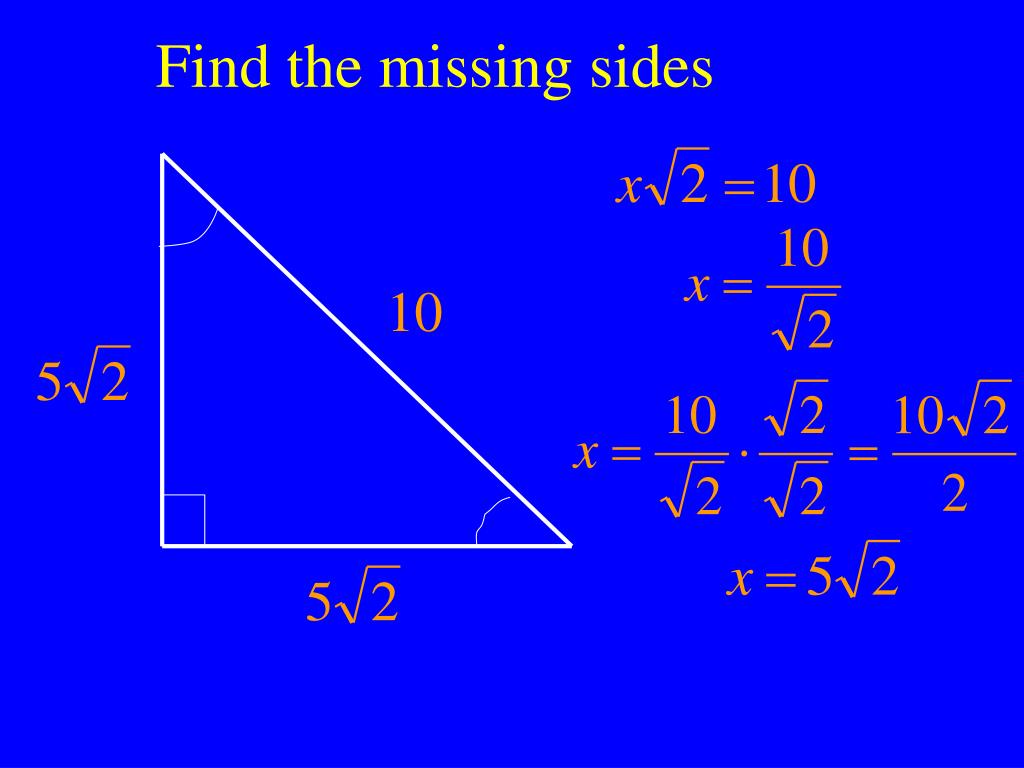
The Pythagorean theorem is a fundamental principle used to find the missing side of a right triangle. It states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed with the formula:

a² + b² = c²

Where:

c is the length of the hypotenuse.
a and b are the lengths of the other two sides.

How to Find the Missing Side of a Right Triangle Using the Pythagorean Theorem?

To find the missing side of a right triangle using the Pythagorean theorem, follow these steps:

Identify the lengths of the two known sides.

Use the Pythagorean theorem formula to solve for the missing side.

Rearrange the formula as needed to isolate the variable representing the missing side.

Can Trigonometric Ratios Help to Find the Missing Side of a Triangle?

Yes! Trigonometric ratios can be used to find the missing sides of not just right triangles but also other types of triangles, given that you have information about at least one angle and one side. The primary trigonometric ratios used are sine, cosine, and tangent.

How to Use Sine, Cosine, and Tangent to Find the Missing Side of a Triangle?

Here's a breakdown of how to use these ratios:

Sine (sin): sin(angle) = opposite side / hypotenuse
Cosine (cos): cos(angle) = adjacent side / hypotenuse
Tangent (tan): tan(angle) = opposite side / adjacent side

To find a missing side:

Identify the known angle and the side you need to find.

Use the appropriate trigonometric ratio based on the angle and the known side.

Rearrange the formula to solve for the missing side.

What is the Law of Sines and How Can it Help Find the Missing Side of a Triangle?

The Law of Sines states that the ratios of the lengths of a triangle's sides to the sines of their opposite angles are equal. It can be expressed as:

a/sin(A) = b/sin(B) = c/sin(C)

This law is particularly useful for non-right triangles. To use the Law of Sines to find a missing side:

Identify two angles and one side or two sides and an angle opposite one of those sides.

Set up the ratio according to the Law of Sines.

Solve for the missing side.

How Can the Law of Cosines Help in Finding the Missing Side of a Triangle?

The Law of Cosines is another formula that helps to find a missing side when you know two sides and the included angle or all three sides. It is stated as:

c² = a² + b² - 2ab(cos(C))

Where:

c is the side opposite angle C.
a and b are the other two sides.

How to Apply the Law of Cosines to Find the Missing Side of a Triangle?

To apply the Law of Cosines:

Identify the two known sides and the included angle or all three sides.

Substitute the values into the Law of Cosines formula.

Solve for the missing side.

What Are Some Real-World Applications of Finding the Missing Side of a Triangle?

Finding the missing side of a triangle has practical applications in various fields:

Architecture: Ensuring accurate dimensions for structural integrity.
Engineering: Designing components that require precise measurements.
Navigation: Using triangulation to determine locations.
Computer Graphics: Calculating dimensions for rendering objects.

Conclusion: Mastering How to Find the Missing Side of a Triangle

In conclusion, knowing how to find the missing side of a triangle is a vital skill that can be applied in numerous fields. By understanding the properties of triangles, utilizing the Pythagorean theorem, and applying trigonometric ratios, the Law of Sines, and the Law of Cosines, you can confidently tackle triangle-related problems. With practice, you will not only improve your mathematical abilities but also gain skills that will be applicable in real-world scenarios.

Article Recommendations

ncG1vNJzZmivp6x7tMHRr6CvmZynsrS71KuanqtemLyue9WiqZqko6q9pr7SrZirq2FktbDDjK2mZp6Zo7FuucisqqKml2LAqrDEZqafZaSntqK6xqWcZ6Ckork%3D

GleamVibe