Understanding .875 In Fraction Form: A Comprehensive Guide
Mathematics can often feel overwhelming, particularly when it comes to understanding decimals and their corresponding fractions. One such decimal that frequently appears in various calculations is .875. Converting decimals to fractions is a fundamental skill that can enhance your mathematical abilities, making it easier to work with numbers in different contexts. In this article, we will explore the process of converting .875 into fraction form, discuss its significance, and answer some common questions related to fractions and decimals. By the end of this guide, you will have a clearer understanding of how to express .875 in fraction form and the broader implications of this conversion in everyday mathematics.
Understanding the decimal .875 is essential for students, professionals, and anyone engaged in activities that require numerical accuracy. Whether you're cooking, budgeting, or working on complex mathematical problems, knowing how to convert decimals to fractions is a valuable skill. The conversion process involves identifying the place value of the decimal and expressing it as a fraction, which can then be simplified if necessary. This article will break down the steps needed to convert .875 to fraction form, offering insights and tips along the way.
As we delve deeper into the world of decimals and fractions, we will address some frequently asked questions regarding the conversion process. What does .875 represent as a fraction? Why is it important to convert decimals to fractions? How can understanding this conversion enhance your mathematical skills? These questions will be answered in detail, equipping you with the knowledge needed to confidently work with decimals and fractions in various scenarios.
What is .875 in Fraction Form?
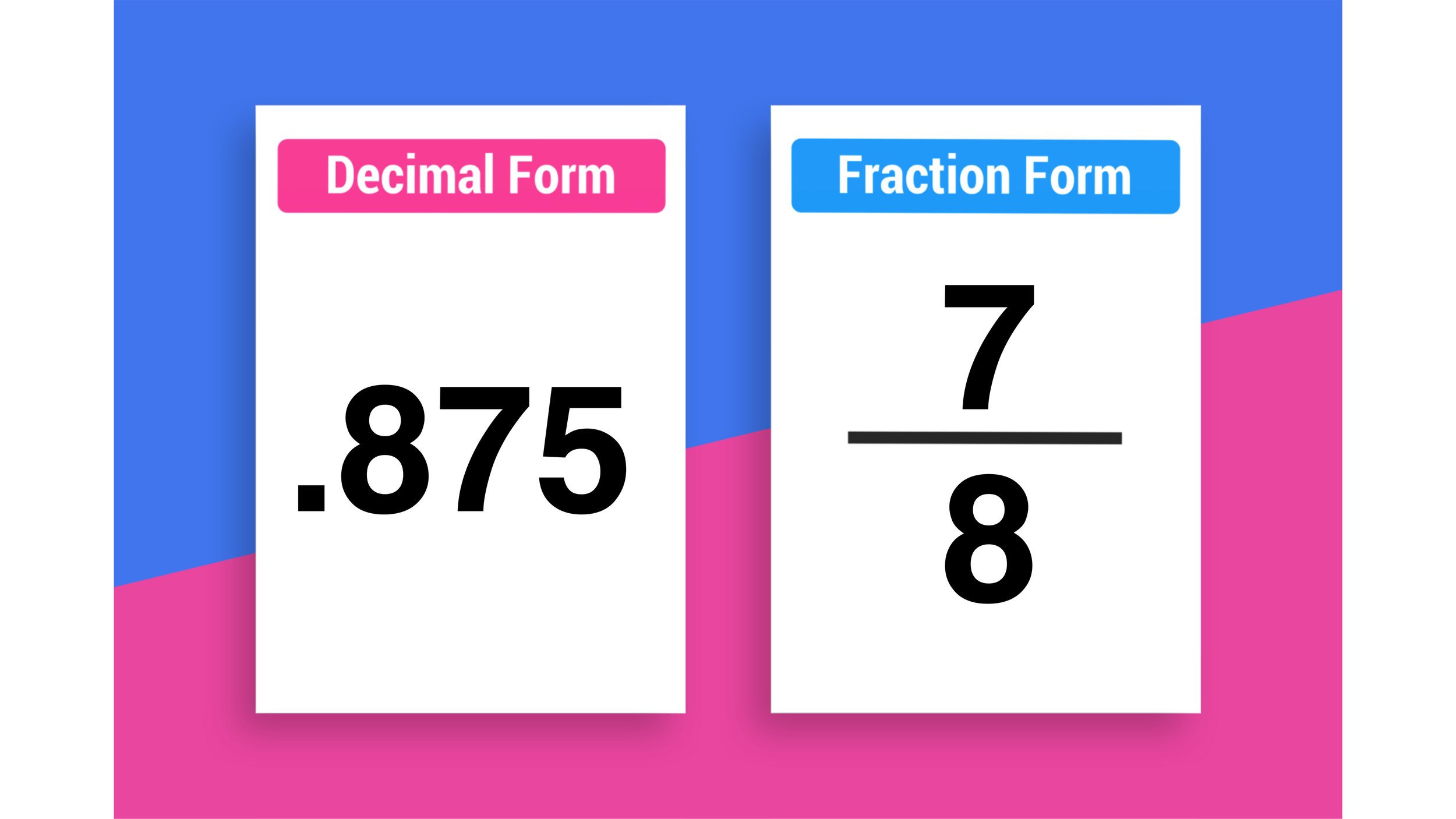
To convert .875 into fraction form, we start by recognizing the decimal's place value. The number .875 consists of three digits after the decimal point, indicating that it can be expressed as:
0.875 = 875/1000
Next, we simplify this fraction. Both the numerator (875) and the denominator (1000) can be divided by their greatest common divisor (GCD), which is 125:
875 ÷ 125 = 7
1000 ÷ 125 = 8
Therefore, .875 in fraction form is:
7/8
Why is Converting .875 to Fraction Important?
Understanding how to convert decimals like .875 to fractions is crucial for several reasons:
- Mathematical Precision: Fractions can represent values more precisely in certain contexts, especially in measurements.
- Ease of Manipulation: Working with fractions can sometimes be easier than working with decimals, especially in algebraic equations.
- Real-World Applications: Many practical situations, such as cooking or construction, often require measurements in fractions rather than decimals.
How Can You Verify the Conversion?
To ensure that your conversion from .875 to 7/8 is accurate, you can perform a simple check by dividing the numerator by the denominator:
7 ÷ 8 = 0.875
This confirms that the conversion is correct, as the decimal form matches the original value.
Are There Other Ways to Express .875 in Fraction Form?
While 7/8 is the simplest form of .875, it can also be expressed in other equivalent fractions. For example:
- 14/16
- 21/24
- 35/40
These fractions, while equivalent to .875, are not in their simplest form. Understanding how to manipulate fractions can aid in various mathematical problems.
How Does .875 Compare to Other Common Decimals?
To provide context, let's compare .875 to other common decimal values:
| Decimal Value | Fraction Form |
|---|---|
| 0.5 | 1/2 |
| 0.75 | 3/4 |
| 0.9 | 9/10 |
| 0.125 | 1/8 |
Each decimal has its unique fraction form, and recognizing these relationships can enhance your numerical understanding.
Can You Use .875 in Real-Life Situations?
Yes, .875 can be found in various real-life scenarios, such as:
- Cooking: When measuring ingredients, you may encounter fractions like 7/8 of a cup.
- Construction: Measurements for materials often require precise fractions.
- Finance: Understanding interest rates and percentages may involve decimal to fraction conversions.
What Are the Benefits of Understanding Fractions?
Grasping the concept of fractions, especially through conversions like .875 in fraction form, offers numerous benefits:
- Improved Problem Solving: A strong foundation in fractions can enhance your ability to solve complex mathematical problems.
- Better Financial Literacy: Understanding fractions can aid in budgeting, saving, and investing.
- Enhanced Communication: Being able to express measurements accurately fosters clearer communication in various fields.
Conclusion: Mastering .875 in Fraction Form
In conclusion, understanding .875 in fraction form, which is 7/8, is a valuable skill that can enhance your mathematical prowess. Whether you're converting decimals for academic purposes or practical applications, mastering this concept offers numerous advantages. By recognizing the importance of such conversions, verifying your work, and applying your knowledge in real-life situations, you can navigate the world of numbers with confidence.
As you continue to explore the relationship between decimals and fractions, remember that practice makes perfect. The more you engage with these concepts, the more intuitive they will become, paving the way for success in your mathematical endeavors.
Also Read
Article Recommendations


ncG1vNJzZmivp6x7tMHRr6CvmZynsrS71KuanqtemLyue9OrsJ6bmKR%2FcnuXcGxmoZ5is7Otwq2gqKZdm7yzuY2hq6ak