Understanding The Squared Symbol: Significance And Applications
The squared symbol, represented as "²", is more than just a mathematical notation; it holds significant meaning across various fields such as mathematics, physics, and even art. This seemingly simple character encapsulates the concept of squaring a number, which is foundational in algebra and geometry. By multiplying a number by itself, this symbol helps us quantify and visualize exponential growth, area calculation, and numerous other applications.
From classrooms to professional settings, the squared symbol is a vital part of our understanding of mathematical relationships. It has evolved to become a universal shorthand for expressing power and magnitude. As we delve deeper into the meaning and implications of this symbol, we discover its relevance not just in mathematics, but also in science, engineering, and even technology.
In the digital age, the squared symbol has found its way into various software and applications, making it easier for users to perform complex calculations with just a click. Whether you're a student grappling with algebra or a professional conducting data analysis, understanding the squared symbol can enhance your ability to interpret and present data effectively.
What Does the Squared Symbol Represent?
The squared symbol "²" represents the operation of squaring a number. When you see this symbol next to a number, it indicates that the number should be multiplied by itself. For example, 3² (read as "three squared") equals 3 x 3, which is 9. The squared symbol is essential in various mathematical contexts, including:
- Calculating areas of squares and rectangles.
- Understanding quadratic equations.
- Expressing relationships in physics, such as force (F = ma) where mass (m) may be squared.
How is the Squared Symbol Used in Mathematics?
In mathematics, the squared symbol is predominantly used in algebra and geometry. Here are some key applications:
1. Area Calculation
The area of a square can be calculated using the formula A = s², where "s" is the length of a side. This means that to find the area, you square the length of one side.
2. Quadratic Equations
Quadratic equations, which are polynomial equations of the form ax² + bx + c = 0, rely heavily on the squared symbol to express their relationships and solutions.
3. Pythagorean Theorem
The squared symbol is also found in the Pythagorean theorem, where a² + b² = c² defines the relationship between the sides of a right triangle.
Why is the Squared Symbol Important in Science?
In scientific contexts, the squared symbol has various applications, particularly in physics. Here are a few examples:
1. Kinetic Energy Formula
The formula for kinetic energy is KE = ½ mv², where "m" is mass and "v" is velocity. The squared symbol indicates that velocity is a crucial factor in determining energy levels.
2. Pressure Calculation
In fluid mechanics, pressure is calculated using the formula P = F/A, where force (F) can sometimes be expressed in squared terms to analyze the effects of area on pressure.
How to Type the Squared Symbol on Different Devices?
Typing the squared symbol may vary across devices. Here’s how you can easily insert it:
- On Windows: Use Alt + 0178 on the numeric keypad.
- On Mac: Use Option + 00B2 in the Unicode input.
- On Smartphones: Access the symbol from the special characters keyboard.
Can the Squared Symbol Be Used in Art and Design?
Interestingly, the squared symbol has also made its way into art and design. Artists sometimes use the squared symbol to convey ideas of balance and symmetry. In graphic design, it can represent a squared-off style or a block-like aesthetic. Here are a few creative applications:
- In logos to create a geometric style.
- In visual art to explore themes of unity and structure.
What Are Some Common Misconceptions About the Squared Symbol?
There are several misconceptions regarding the squared symbol that need clarification:
1. Is the Squared Symbol Only for Positive Numbers?
No, while squaring a negative number results in a positive value, the squared symbol can be applied to both positive and negative numbers.
2. Does Squaring Always Result in a Larger Number?
This is true for numbers greater than one; however, squaring a fraction (between 0 and 1) will yield a smaller number.
Conclusion: Embracing the Squared Symbol
The squared symbol is a powerful tool in mathematics, science, and art. Its ability to convey complex ideas in a simple manner makes it an essential part of our educational and professional toolkit. Understanding its applications allows us to appreciate the depth and breadth of mathematical concepts and their relevance to our daily lives. As we continue to explore the world around us, the squared symbol will undoubtedly remain a constant companion in our quest for knowledge.
Also Read
Article Recommendations

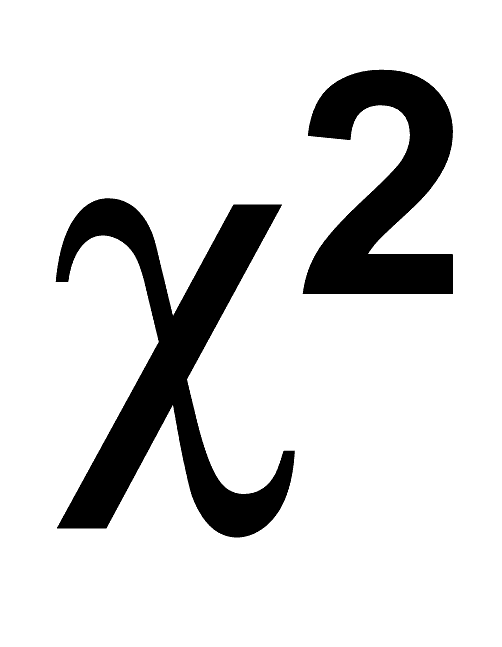

ncG1vNJzZmivp6x7tMHRr6CvmZynsrS71KuanqtemLyue9WiqZqko6q9pr7SrZirq2JkwLLBwKucnWWjrrqju8tnn62lnA%3D%3D